|

|

|
Cube Tube
Video and documentation to help you catch the vision of working like a mathematician. A mathematician's work begins with an interesting problem. Explore:
- Principles
- Problems
- Teaching Craft
View on screen, or download if you wish, but please acknowledge the source.
|
|

LIBRARY
Click a link to find information about the video and its play link.
DIY Workshop Videos
We provide the workshop content.
You provide the workshop leader.
|
|
|
Find our Cube Tube Channel at: https://www.youtube.com/@cubetubemaths



|

|
Workshop:
Aiming High? Dig Deeper
Inspired by a staircase of natural numbers and in the context of learning to work like a mathematician in fascinating, captivating and absorbing classrooms, this DIY video workshop is for teachers K-12 who suspect students might learn more by digging deeper into a rich learning experience, than by moving on to the next something. Mathematics does not have to be taught the way it always has been. Using a maths journal is integral to the workshop.
The local leader arranges preparation, encourages discussion before, during and after the session and manages the use of time during the workshop. The video provides stimulus content. There are pauses for activity and discussion. Add your own pauses too as appropriate. Allow at least 1 hour for the session. This video can be used in sections over several sessions.
Preparation
Room
- Teachers in pairs grouped at tables.
- Computer, screen, sound system, whiteboard
Printing
- Guide to Video Sections & Content - 1 page
- Investigation Guide - 1 page
- Isometric Paper - 1 page
- 1cm Graph Paper - 1 page
- Working Mathematically - 2 pages
- Learning Features - 1 page
Teachers
- Paper and pen / pencil to use as a Maths Journal.
- At least 15 linking cubes or wooden cubes per pair
Click an image to begin the video. (Time ... 37:45)
|
|



|

|
Workshop:
A School Without Books
A teacher once commented in a workshop that:
A school without tasks is like a school without books.
This DIY workshop gently leads participants through activities which draw out the features of using hands-on problem solving tasks which might stimulate a person to make this comparison. Along the way the structure, navigation tools, content depth and teaching craft focus of Mathematics Centre are explored through the investigations Truth Tiles 2 (Years 2 - 10) and The Mushroom Hunt (Years 4 - 10). The workshop begins by imagining the possible educational losses in a school without books and ends by imagining the possible educational gains in a school with tasks.
The local leader arranges preparation, encourages discussion before, during and after the session and manages the use of time during the workshop. The video provides stimulus content. There are pauses for activity and discussion. Add your own pauses too as appropriate. Allow at least 1 hour for the session.
Preparation
Room
- Teachers in pairs grouped at tables.
- Computer, screen, sound system, whiteboard
Printing
- Working Mathematically - 2 pages
- Learning Features - 1 page
Teachers
- Paper and pen / pencil
- Truth Tiles 2
One sheet of paper between 2 folded into 8 sections, sharply
creased and torn into 8 tiles.
Each tile records one of 3, 4, 5, 6, 7, +, -, =
(Some leaders prefer to have teachers prepare these tiles during the
introductory moments of the workshop.)
- The Mushroom Hunt
For each group of 3 or 4, three Poly Plug red boards and
6 paper plates, if available, or 1 container with about 70
counters or cubes and 6 pieces of paper.
- Digital equipment will be in the way in a person to person
session.
- It's essential in a remote session but best if the leader shows
the video on a shared screen.
Click an image to begin the video. (Time ... 31:16)
|
|

|

|
Workshop:
Engineering 'aha' Moments in Number
Calculating Changes is professional development supporting K-6 teachers to develop children's number sense and skills beyond what is normally expected for the age. In the context of learning to work like a mathematician, the key is engineering 'aha' moments in number more often for more children. This DIY video workshop invites your teachers to begin considering what might be learnt from the decades of experience gathered by Calculating Changes teachers.
The local leader arranges preparation, encourages discussion before, during and after the session and manages the use of time during the workshop. The video provides stimulus content. There are pauses for activity and discussion. Add your own pauses too as appropriate. Allow at least 1 hour for the session.
Preparation
Room
- Teachers in pairs grouped at tables.
- Computer, screen, sound system, whiteboard
Printing
- Working Mathematically - 2 pages
- Learning Features - 1 page
Teachers
- Paper and pen / pencil
- 1 Poly Plug per pair
If you don't have Poly Plug print this Poly Plug Frame and use counters.
- 1 calculator per pair
- 1 dice per pair
- Digital equipment will be in the way in a person to person
session.
- It's essential in a remote session but best if the leader shows
the video on a shared screen.
Click an image to begin the video. (Time ... 31:47)
|
|


|

|
Workshop:
Multiplication Journey
Beginning with building and exploring equal rows of objects (arrays) at a young age, learning grows into single digit multiplication (times tables), short multiplication, long multiplication, division as working multiplication backwards and on into algebra as a natural extension of partitioning arrays. This DIY video workshop invites your teachers to build teaching craft from concrete materials and diagrams (semi-concrete) to symbols and generalisation within differentiated classrooms and across the school, all within the context of learning to work like a mathematician.
The local leader arranges preparation, encourages discussion before, during and after the session and manages the use of time during the workshop. The video provides stimulus content. There are pauses for activity and discussion. Add your own pauses too as appropriate. Allow at least 1 hour for the session.
Preparation
Room
- Teachers in pairs grouped at tables.
- Computer, screen, sound system, whiteboard
Printing (used in the session review)
- Working Mathematically - 2 pages
- Learning Features - 1 page
Teachers
- Paper and pen / pencil
- 1 Poly Plug per person or 30 small cubes or similar per pair
- 2 drinking straws or similar per person
- Digital equipment will be in the way in a person to person
session.
- It's essential in a remote session but best if the leader shows
the video on a shared screen.
Click an image to begin the video. (Time ... 16:41)
|
|


|

|
Workshop: Poster Problem Clinic
This DIY video provides a framework for a teachers' workshop guided by a local leader. Allow at least one hour for the workshop. A Poster Problem Clinic is a technique for helping students learn to work like a mathematician when tackling a problem. A Professor Morris Puzzle is used as the example in the workshop. The concept is adaptable to all levels. It is based around Polya's stages of:
- Read & understand the problem.
- Plan a strategy to start the problem.
- Carry out the plan.
- Check the result.
The local leader arranges preparation, encourages discussion before, during and after the session and manages the use of time during the workshop. The video provides stimulus content. There are pauses for activity and discussion. Add your own pauses too as appropriate.
Preparation
Room
- Teachers in pairs grouped at tables.
- Computer, screen, sound system, whiteboard
Printing
- Working Mathematically - 2 pages - start of session
- Learning Features - 1 page - near end of session
- Follow Up Resources - 1 page - close of session
Teachers
- Paper and pen / pencil
- Digital equipment will be in the way in a person to person
session.
- It's essential in a remote session but best if the leader shows
the video on a shared screen.
Click an image to begin the video. (Time ... 23:50)
|
|

|

|
Staircase Maths: in less than one minute
Everyone can spare a minute to watch this video. Be stunned by the breadth and depth of mathematics learning captured in the image of a staircase of blocks. Follow up for yourself or with a partner, small group or collegiate team using the DIY workshop Aiming High? Dig Deeper.
Click the image to begin the video. (Time ...00:54)
|
|

|

|
Workshop: The Frog Pond
This DIY video is a workshop starter. Perhaps you can use it in a faculty meeting to generate a refreshing discussion about learning features and what you value in mathematics teaching. The content is consistent with any curriculum that includes chance and data and the investigation has been successfully used in classes from K to 8. But it is also an unfinished workshop. You will need to follow the investigation beyond the video to experience some of its mathematical surprises.
Does the investigation have a place in middle or upper secondary school?
Preparation
Room
- Teachers in pairs grouped at tables.
- Computer, screen, sound system, whiteboard
Printing
- Working Mathematically - 2 pages - to enrich discussion
Teachers
- Paper and pen / pencil
- Poly Plug or counters
- Paper plate or similar 'pond'
- Dice
Click a photo to begin the video. (Time ... 6:02)
|
|

|

|
Adventure of the Sphinx
Dani and Alex introduce you to the amazing investigation by Pam McGifford's Year 8 class at Cressy District High School into the remarkable properties of a shape called the Sphinx. You will discover unexpected connections between shape, pattern, measurement and algebra and you will be stunned by the competence and confidence of these young people who are Learning to Work like a Mathematician. Perhaps the most amazing thing of all - or perhaps not if you have ever been captivated by mathematics - is that the students did most of their problem solving, as a group, on their own during Mrs. McGifford's absence. Pam had introduced the problem to them using Sphinxes made by her husband. When she returned to school and saw what her children had achieved and the confidence and enthusiasm with which they expressed it, she encouraged them to publish their work 'just like a mathematician would'.
- Learn more about Task 166, Sphinx in its cameo and the Sphinx Album.
- Maths300 members can find out more about this investigation in Lesson 25, Sphinx.
Click the photo to begin the video. (Time ... 8:40)
|
|

|

|
A Lockdown Learning Star
Jojo, Year 6, was doing school at home. Her screen presented questions about line symmetry of polygon shapes. She decided to make them from newspaper tubes (as in the Maths At Home activity below) ... then she sent us a video. It's wonderful. We added a little music and titles and included it in the activity notes.
Click the photo to begin the video. (Time ... 2:56)
|
|

|

|
An Ocean of Possibilities
First presented through the web site of the Association of Teachers of Mathematics (ATM), UK, this video introduces an alternative vision of mathematics education that is driven by the Working Mathematically Process identified by mathematicians. The video was produced in conjunction with the article An Ocean of Possibilities, published in Mathematics Teaching 217, March 2010, by ATM.
- Learn more about Task 216, Square Pairs.
- Maths300 members can find out more about this investigation in Lesson 140, Square Pairs.
Click the photo to begin the video. (Time ... 2:15)
|
|
|

|
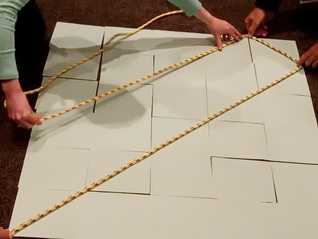
Billiard Ball Bounces
Billiard Ball Bounces is a Maths300 lesson which doesn't grow from a task. But as Matt Skoss shows us in this video made at a professional development session, it can start very practically on the floor. All you need is a set of cards about 20cm square and a length of coloured cord. The cards are used to make a 'billiard table' of any size, in this case 6 x 5, and the cord traces the path of a ball hit from the bottom left pocket. The challenge is to predict the number of bounces before the ball 'drops' into a corner pocket.
- Maths300 members can find out more about this investigation in Lesson 55, Billiard Ball Bounces.
Click the photo to begin the video. (Time ... 1:13)
|
|

|

|
Bob's Buttons
Bob's Buttons has many solutions and each one has to satisfy two conditions - a solution must be able to share between 4 and have 2 left and share between 5 and have 1 left. Jamie and Jack, Year 5, Ashburton Primary School, show how satisfying one condition can be transformed into satisfying the other.
- Learn more about Task 123, Bob's Buttons.
- Maths300 members can find out more about this investigation in Lesson 10, Bob's Buttons.
Click the photo to begin the video. (Time ... 0:36)
|
|

|

|
Cars In A Garage 1
Jessica & Madeleine, Year 4 at Ashburton Primary School, Victoria, explain how they know that three cars can be parked in three garages in only 6 ways. The critical element of the explanation is the recognition that two cars can only be parked two ways in two garages - this way or that way.
- Learn more about Task 2, Cars In A Garage.
- Maths300 members can find out more about this investigation in Lesson 128, Cars In A Garage.
Click the photo to begin the video. (Time ... 0:17)
|
|

|

|
Cars In A Garage 2
Compare Cars In A Garage 1 with this explanation from William also in a Year 4 class at Ashburton Primary School, Victoria. He also understands the element critical to all combination theory challenges.
- Learn more about Task 2, Cars In A Garage.
- Maths300 members can find out more about this investigation in Lesson 128, Cars In A Garage.
Click the photo to begin the video. (Time ... 0:37)
|
|
|

|
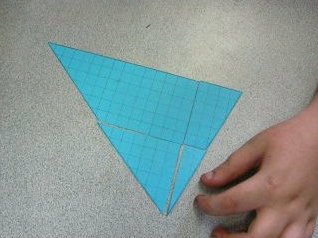
Changing the Triangle
A child in Year 5/6 at Rangebank Primary School, Victoria, explains how to change a triangle into a rectangle.
- Can your students reconstruct the vital unspoken elements of the demonstration?
- Can your students extend the 'aha' moment implied by the video to discover how to calculate the area of triangle?
This Investigation Guide might help. The mathematical content in the investigation is embodied in Task 187, Triangle Area, from the Mathematics Task Centre and Lesson 44, Area of a Triangle, from Maths300. The Maths300 lesson also supplies software.
Click the photo to begin the video. (Time ... 0:11)
|
|
|

|
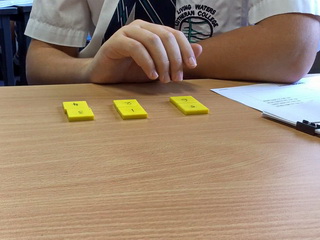
Does This Solve 'A Stacking Problem'?
A student at Living Waters Lutheran College is challenging us to decide if these moves are a solution to Task 149, A Stacking Problem. You might like to introduce your students to the problem, let them play for a while, then ask them to answer the student's question: Do my moves solve the problem?
- If yes, explain why.
- If no, explain why not?
- Do you think mathematicians make mistakes?
Find out more about Task 149, A Stacking Problem, in its Task Cameo, which includes an Investigation Guide supporting students to find ways to build a solution strategy.
Click the photo to begin the video. (Time ... 0:41)
|
|

|

|
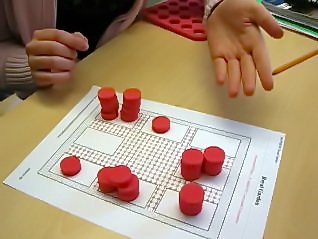
Four & Twenty Blackbirds
In this Year 7 class at Trädgårdsstadsskolan, Sweden, the teacher explains the problem at a central table using a fish bowl strategy. The table is a courtyard and around the perimeter there are eight feeding platforms, one at each corner and one at the midpoint of each side.
Twenty-four blackbirds land so that there are nine along each side. How are they arranged?
Video 1 shows that this story shell has captured the students' interest. The conversation is in Swedish.
Video 2 is later in the lesson when Sofia, Maria & Annika explain, in English, how they can find every solution.
Click a photo to begin the video. (Time ... Video 1 = 0:32, Video 2 = 1:57)
|
|

|

|
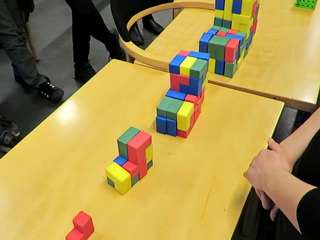
Growing Tricubes
This class of trainee secondary teachers at Högskolan Malmö had discovered that Tricubes can grow reasonably easily by powers of 2. They had made Size 1, Size 2 and Size 4 by working together.
- But what happened to Size 3?
- If there is a Size 3 could we predict the number of Tricubes to make it?
- If we have that number, is it possible to make it?
Video 1 shows the struggle to try to build Size 3.
Video 2 is just a few minutes later when it has been achieved.
But that just leads to asking more questions...
Click a photo to begin the video.
(Time ... Video 1 = 1:43, Video 2 = 0:47)
|
|

|

|
Haberdasher's Problem
The original puzzle was to dissect an equilateral triangle to make pieces that could be rearranged as a square. That puzzle was solved by Henry Dudeney in the late 1800s and these Swedish teacher trainees have the pieces that result from the dissection. However, it is clear that the challenge of using these to make both the square and the equilateral triangle is non-trivial. When achieved, it is the tip of the iceberg which then interests students in the original challenge and develops into a history of mathematics lesson requiring significant geometry.
- Learn more about Task 146, Haberdasher's Problem.
- Maths300 members can find out more about this investigation in Lesson 86, Haberdasher's Problem.
Click the photo to begin the video. (Time ... 0:31)
|
|
 
Video 1 Video 2

Video 3
|

|
Hearts & Loops 1, 2 & 3
Nick and Owen, Year 4, Ashburton Primary School, are learning to work like a mathematician. First they become interested in a problem, in this case a problem from topology related to open and closed curves. If they can separate the heart from the loop, then both curves were never closed.
For a mathematician, solving a problem is not enough. A mathematician must be able to explain the solution.
Nick shows us that he can separate the pieces (Video 1). Owen shows us that he can reconnect them (Video 2). Then Owen introduces Nick (Video 3) who, with his hands behind his back, teaches the teacher how to separate the pieces.
Click a photo to begin the video.
(Time ... Video 1 = 0:23, Video 2 = 0:11, Video 3 = 1:18)
|
|

|

|
How Does it Feel to Work Like a Mathematician?
A mathematician's work begins with an interesting problem. Kathy and Steve are certainly interested in the problem Squound which was presented to them in a workshop. Join them in the middle of their muddle to get a sense of what your students need to experience if they are ever to learn how to work like a mathematician. Then investigate the problem for yourself - it will take a while - and come back to watch the video again to reflect on how it feels to work like a mathematician. Consider too the features which have made it more likely that these teachers would be fascinated, captivated and absorbed by Squound.
- Learn more about Task 139, Squound.
Click the photo to begin the video. (Time ... 4:25)
|
|


|

|
In Between Time & Wait One Minute
These two videos are part of the learning involved in the Maths At Home activity titled In Between Time. The first introduces the activity and the second offers a closing challenge. It is essential that the learner has an analogue clock which they can manipulate and preferable that they have a partner. The initial video demonstration invites students to investigate the interconnectedness of the hour and minute hands. The activity moves on to a thirty minute difference from a given time, a challenge which has two answers each time. The second video concentrates on the second hand and its relationship to minutes.
The MAH form of In Between Time derives from Task 25, with the same name, which itself is a partner to Task 22, Time Together.
Click a photo to begin the video.
(Time: Video 1 ... 4:24, Video 2 ... 2:49)
|
|

|

|
Introducing Bob's Buttons
Bob's Buttons is introduced through a game using pretend people in a pretend school ground. The presenter models how the learners can play the game for themselves, then invites them to dig into the investigation at the Maths At Home (MAH) site to find the question Bob asked which turned the game into an amazing investigation. The video with its on-going development at MAH offers many opportunities to reconsider mathematics pedagogy and to recognise the value of mathematics learning being framed in the concept of learning to work like a mathematician.
Click the photo to begin the video. (Time ... 6:18)
|
|
|

|
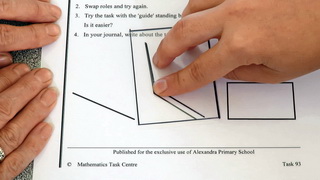
Leading The Blind
Kristen and Jo, teachers at Alexandra Primary School, investigate Task 93, Leading The Blind, from the eTask Package as part of a professional development day entitled Learning to Work Like a Mathematician. Student A guides Student B (who is 'blind') to place a copy of a shape exactly on top of its original. The focus of this task is encouraging visual imagery and the recognition and refinement of mathematical language.
Listen to the video a second time and make two lists - one for natural language used and one for mathematical language. Discuss.
Click the photo to begin the video. (Time ... 1:23)
|
|
 
Video 1 Video 2
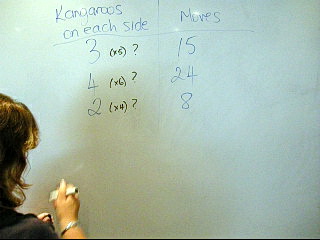 
Video 3 Video 4

Video 5
|

|
Lunching With Jumping Kangaroos
Three secondary schools decided they could best support change in their maths departments by funding long term professional development for key teachers. They chose a 6 day program, Engineering 'aha' Moments in Algebra, from Mathematics Centre, and two or three teachers from each school to participate. This video sequence shows the teachers choosing to eat their lunch while working together on the Jumping Kangaroos task. Perhaps evidence in itself of the success of the course.
- Video 1: Playing with the initial problem (Time ... 2:30)
- Video 2: Success, peer teaching, what happens if...? (Time ... 6:33)
- Video 3: Collecting class data, developing a hypothesis (Time ... 0:17)
- Video 4: Discussion, consensus (Time ... 2:33)
- Video 5: Oh no! there's more. (Time ... 0:22)
Learn more about
Click a photo to begin the video.
|
|

|

|
Making a Maths Mat with Matt
A 'how to' video in which Matt is joined by Eleni and Alex to make a 4.8m x 7.2m Maths Mat for Eleni's school. A grid of fifty 65cm squares on one side and a sorting network on the other; all done by three people in less than three hours. Learn how to make your own mat, discover the importance of whole body involvement as a learning feature and know where to find activities that will get you started.
Click the photo to begin the video. (Time ...08:34)
|
|

|

|
Maths on a Mat: 3 Generations
Eleni Pilafas, Matt Skoss and Doug Williams represent three generations of teachers who have used the Maths Mat to encourage learning mathematics through whole body involvement. At the 2024 annual conference of The Mathematical Association of Victoria they jointly presented a workshop using a double-sided mat to explore activities from K-10. The video presents extracts from this workshop.
Click the image to begin the video. (Time ...11:20)
|
|

|

|
Maths on a Mat with Mat
A snapshot of activities from Matt Skoss's workshop at the 2023 December Conference of the Mathematical Association of Victoria. Whole body involvement in learning mathematics. View the video and follow up with many more activities and how to make your own mat in this reference.
Click the photo to begin the video. (Time ...0:57)
|
|

|

|
Making & Breaking Rectangles
Paul Haenen's Year 3/4 at Loxton North Primary School are on a Multiplication Journey. Today they are exploring different arrays for the same number and recording what they find. They are using bottle tops as their equipment and are very willing to talk about the mathematics they are experiencing. It's all about equal rows ... and rows going across your tummy, just like they do for an actor on stage.
Making & Breaking Rectangles is a member activity from Calculating Changes.
Click the photo to begin the video. (Time ... 2:40)
|
|

|

|
Move Around
Jamie Kemp, St. Francis of Assisi Primary School, Calwell, explores Move Around, an activity from Calculating Changes with Year 6 and Year 3 students. The children learn heaps about numbers on the number line, place value - even decimals - and Jamie learns heaps about teaching. This could be the best 11 minutes of professional development you have ever experienced.
Click the photo to begin the video. (Time ... 10:54)
|
|

|

|
Multiplication with Poly Plug
Back Story
About 3 months before making the video, this Year 9 student went with her mum to visit the grandparents for Sunday lunch. When the adult conversation lost her interest, she wandered off to the bedroom where the grandchildren stay over. She found Poly Plug in the toy cupboard and happily sat on the carpet and began playing. She drifted into building walls, then noticed patterns and just before leaving invited the adults to look at what she had discovered.
The day before the video was made the grandfather received a text. When you pick me up after school tomorrow can you bring the Poly Plug. I want to make a video for my algebra class.
Click the photo to begin the video. (Time ... 09:19)
|
|

Video 1
 
Video 2 Video 3
|

|
My Professional Learning 1, 2 & 3
In three short videos three teachers explain what they learnt in a full day course titled Learning to Work Like a Mathematician.
The description of the course was:
For a mathematician to start work they must first have an interesting problem. Their work involves applying reasoning, questioning, justification and communication processes in the pursuit of a solution. Therefore when we view the school curriculum as learning to work like a mathematician, we begin with problems - genuine problems with unknown answers, not repetitive exercises with expected answers - and select teaching craft designed to fascinate, captivate and absorb learners.
The course was included in the 2015 Annual Conference of the Association of International Schools of Africa in both a primary and a secondary version. These videos were made voluntarily at the end of the Primary day.
Click a photo to begin the video.
(Time ... Video 1 = 1:09, Video 2 = 0:13, Video 3 = 1:07)
|
|

|

|
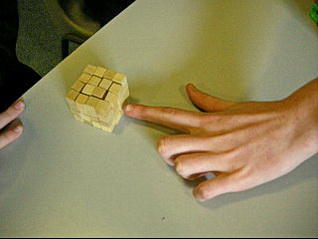
Painted Cubes 1
Watch and listen as a group of Year 8 girls from Settlebeck High School, England, explore how many unit cubes have 0, 1, 2, and 3 faces painted if their 4x4x4 cube has all its outside faces painted.
- Learn more about Task 160, Painted Cubes.
- Maths300 members can find out more about this investigation in Lesson 38, Painted Cubes.
Click the photo to begin the video. (Time ... 3:08)
|
|
|

|
Painted Cubes 2
A group from the same class as Painted Cubes 1 who use different equipment and show evidence of multiplicative thinking. However, explaining that thinking to each other is a bit of a problem for some in the group. In the end the teachers asks if he may show, rather than tell the explanation. But then the video had to stop. What might he have been intending to do?
Click the photo to begin the video. (Time ... 2:14)
|
|
|

|
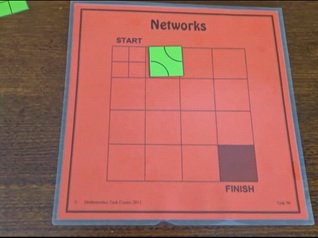
Piper Explores Networks
Piper, just five years old, is already working like a mathematician. Let her teach you about Networks, then follow up with the web reference provided in the video and listed here.
All the materials you need to reproduce this activity are provided in the link.
Click the photo to begin the video. (Time ... 6:20)
|
|

|

|
Potato Olympics 1
Anne Mullans, St. Patrick's Primary School, Murrumbeena, produced this inspirational video to stimulate their Potato Olympics adventure in 2008. That year also happened to be the official human year of the potato, but to potatoes every year is the year of the potato, so the video remains very relevant to any Olympic year. Use it as is, or get inspired and create your own 'video-duction' to this event.
Potato Olympics derives from the work of one Swedish teacher recorded in this article, Potatis Matematik, published in The Classroom Connection, Vol.4 No.2, April - June 1996. From there, various teachers from many places have played with the idea of using potatoes as a concrete teaching aid and, somewhere, the idea of Potato Olympics, as described in Anne's video was born. Maths300 Lesson 67, has collected many of these ideas and includes fabulous Classroom Contributions from Year 7, Luther College, Victoria ... Year 5/6 Kingston, Tasmania ... and Fregon School in very far outback South Australia.
Click the photo to begin the video. (Time ... 1:14)
|
|

|

|
Potato Olympics 2
Created and mounted to YouTube by Chairo Christian School, Victoria, this video provides insight into one school's interpretation of the Potato Olympics challenge. Also, Sue Gibson, Mount Dandenong Primary School, Victoria, has written with equal excitement about her experiences at:
Click the photo to begin the video. (Time ... 3:41)
|
|

|

|
Potato Olympics Oath
On behalf of their decorated potatoes, Mel Ferguson's Year 5 class, Mt. Carmel School, Yass, New South Wales, swear their Potato Olympics Oath and begin another day of competition. You can see some of their spudletes in action in Potatoes in Action.
Also, Leah Taylor, St. Anthony's School, Wanniassa, ACT, confirms the involvement and learning Mel experienced in this PowerPoint summary of her Potato Olympics experiences.
Click the photo to begin the video. (Time ... 0:27)
|
|

|

|
Princess Catharina's Trinket Chest
Inspired by the trinket chest on her dressing table, Princess Catharina teaches us the most important concept in understanding fractions - that fractions make sense when you know the whole. The video begins with visual learning supported by written language and develops into a problem solving experience for a small group or whole class.
Princess Catharina has also shared her love for her trinket chest and working like a mathematician in Maths At Home investigation:
Click the photo to begin the video. (Time ... 2:00)
|
|

|

|
Seats on the Bus
Junior Primary children at Loxton North Primary School are on a Multiplication Journey. Today their teacher has set out the children's chairs in an array facing forward, just like rows of seats on a bus. The children learn about equal rows of people on the bus and transfer their learning to a model of the context. You can see and sense groundwork developing for multiplication, the commutative law, factors, multiples, primes and times tables.
The video was developed in the context of a 6 Day Professional Development course which drew significantly from Calculating Changes for many of its activities.
- Use the Calculating Changes Content Finder to explore other multiplication activities for all primary years. Some are only available to members.
- Review Calculating Changes Professional Development courses.
Click the photo to begin the video. (Time ... 06:20)
|
|

|

|
Teaching Craft with 4 Arm Shapes
Two colleagues explore a little teaching craft using Task 154,
4 Arm Shapes. The video was made by the Association of Teachers of Mathematics, UK, and was first published in Mathematics Teaching 223, July 2011.
Update 2025: Unfortunately this video is no longer available on the ATM site. Please explore the video An Ocean of Possibilities above, which introduces the teaching craft underpinning the missing video. Then consider following up with these links:
- Learn more about Task 154, 4 Arm Shapes.
- Maths300 members can find out more about this investigation in Lesson 40, 4 Arm Shapes.
|
|

|

|
Times Tables Torture
Supporting the Maths At Home activity of the same name, the video demonstrates how to use the Times Tables slides provided in the activity to create your own slide show or video. This could be the scariest way of practising times tables that has ever been invented. The viewer is expected to learn by taking a taking a Times Tables Torture Test themselves. Are you game?
The final sequences offer practical ways to practise remembering the few you don't know and a practical, hands on representation of every necessary times table.
Click the photo to begin the video. (Time ... 07:50)
|
|
|

|
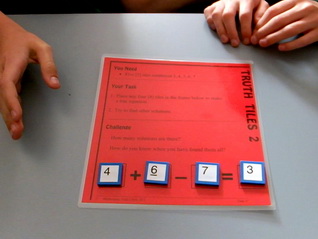
Truth Tiles 2
Truth Tiles 2 begins with a simple equation, namely:
 + +  - -  = = 
Using just the digits 3, 4, 5, 6, 7 we are asked to find solutions. Blair and Alexander, Year 5, Ashburton Primary School, using tasks for the first time, offer a perceptive explanation that not only shows some solutions, but suggests an approach that will help to find all solutions.
- Learn more about Task 17, Truth Tiles 2.
- Maths300 members can find out more about this investigation in Lesson 168, Truth Tiles 2.
Click the photo to begin the video. (Time ... 2:52)
|
|

|

|
Working Like A Mathematician
Eric The Sheep is used as an example of what it means to work like a mathematician. The video, produced for the ATM YouTube channel in May 2012, emphasises becoming interested in a problem, collecting and organising data and making and testing hypotheses.
Update 2025: Unfortunately this video is no longer available on the ATM site. Please explore the video An Ocean of Possibilities above, which introduces the teaching craft underpinning the missing video. Then consider following up with these links:
- Learn more about Task 45, Eric The Sheep.
- Maths300 members can find out more about this investigation in Lesson 17, Eric The Sheep.
|
|



|

|
Working Mathematically with Viruses
These three videos are embedded in the activity notes for a series of investigations titled Working Mathematically with Viruses. They will have most meaning if viewed from within that context (see below).
All our work is based around the concept of learning to work like a mathematician, rather than just practising the skills of a mathematician, so in this activity the focus is on:
- developing and interrogating a model of virus spread
- the effect of public health actions on the spread
- and the potential of a vaccine to control the virus more permanently if enough people accept the invitation to immunise.
The model is presented through Excel programming by Dr. Ian Lowe and a variety of teaching craft, including these videos.
- Part 1: Virus Basics (Time: ... 5:47)
- Part 2: Recovery (Time: ... 5:13)
- Part 3: Effect of Vaccination (Time: ... 4:23)
Learn more about the mathematics and the teaching craft at the Maths at Home activity:
Click a photo to begin the video.
|
|

|

|
Yellow to Blue
Bella, Emma, Amy & Beatrice, Year 6, Camberwell Primary School, describe their insightful algorithm for changing yellow to blue. The problem uses plugs with a different colour on each side. The challenge is to begin with four yellow plugs and by turning over all except one on any turn, to change them all to blue. You might like to try it yourself first because it isn't as easy as the girls make it look. Instead of plugs use four scraps of paper with a cross on one side of each.
- Learn more about Yellow to Blue which derives from Task 132, Red To Blue.
- Maths300 members can find out more about this investigation in Lesson 127, Red To Blue.
Click the photo to begin the video. (Time ... 0:54)
|
|
Cube Tube on You Tube

More from Mathematics Centre
|